Bernoulli and binomial relations
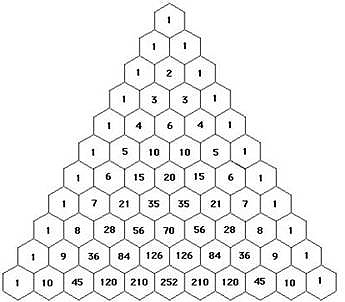
Two sets of numbers that appear all over mathematics are the sequence of prime numbers and the binomial coefficients (“Pascal’s Triangle” below). An interesting connection between the two was discovered in 1913 by A. Fleck who found that if one picks a prime number p and then takes the alternating sum of the elements of the t-th row of Pascal’s triangle separated by a distance p, then this alternating sum is divisible by p to the power of u, the largest number with (p-1)u ≤ t-1. This property is known as Fleck’s congruence. For example, the bottom row of the part of Pascal’s triangle given below corresponds to t =10 and consists of the numbers 1,10,45,120,210,252,210,120,45,10,1. When p =3, and t =10, the inequality (p-1)u ≤ t-1 becomes 2u ≤ 9, so u = 4 and the 3 alternating sums of row elements 3 spaces apart are 1-120+210-10=81, 10-210+210-10=0 and 45-252+45=-162. The numbers 81, 0 and -162 are called Fleck numbers and, sure enough, each of these numbers is divisible by 3^4 = 81 and so satisfies Fleck’s congruence.
Recently connections have been made via matrix theory that link the Fleck numbers to the Bernoulli numbers, which themselves are closely connected to the prime numbers and also the Riemann zeta function (see references below).
 |
References
|
