Representations of integers

Can every reasonably large number be written as a sum of three prime numbers, or four perfect squares, or nineteen fourth powers (1 to 18 cannot be sums of nineteen fourth powers unless you count zero)? If the number of solutions is probably large, exponential sums such as Weyl sums are used. Sieve methods may help too.
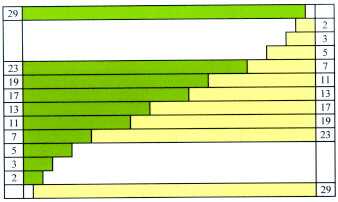
Representations of 30 as a sum of two primes:

References

• M. N. Huxley, One-sided sifting density hypotheses in Selberg's sieve, Number theory (Turku, 1999), de Gruyter, Berlin, 2001, 105–114.
• G. R. H. Greaves, G. Harman, M. N. Huxley (ed), Sieves, Exponential Sums, and their Applications in Number Theory, Cambridge 1997.
• R. C. Vaughan, The Hardy-Littlewood Method, Cambridge 1997.